Planar VTOL

The following figure describes the variables and parameters of the model:

Fig1. - PVTOL schematics.

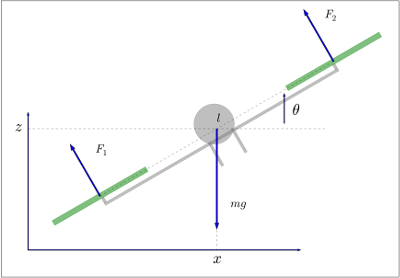
Generalized coordinates

$$\vect{q} =\begin{pmatrix}x&z&\theta\end{pmatrix}^T$$

Kinetic energy

$$T = \frac{1}{2} m(\dot{x}^2+\dot{z}^2) + \frac{1}{2} J \dot{\theta}^2$$

Potential energy

$$V = mgz$$

Lagrangian

$$\begin{align} L &= T-V \\ L &= \frac{1}{2} m(\dot{x}^2+\dot{z}^2) + \frac{1}{2} J \dot{\theta}^2 - mgz \end{align}$$

$$ \begin{cases} \frac{\partial{L}}{\partial{x}} = 0 \\ \frac{\partial{L}}{\partial{z}} = - m g\\ \frac{\partial{L}}{\partial{\theta}} = 0 \\ \end{cases} $$

$$ \begin{cases} \frac{\partial{L}}{\partial{\dot{x}}} = m\dot{x} \\ \frac{\partial{L}}{\partial{\dot{z}}} = m\dot{z} \\ \frac{\partial{L}}{\partial{\dot{\theta}}} = J \dot{\theta} \end{cases} $$

Lagrange Equations

*

$$\frac{d}{dt}\left( \frac{\partial{L}}{\partial{\dot{x}}} \right) - \frac{\partial{L}}{\partial{x}} = F_x$$ $$m\ddot{x} = -(f_1+f_2) \sin{\theta}$$

*

$$\frac{d}{dt}\left( \frac{\partial{L}}{\partial{\dot{z}}} \right) - \frac{\partial{L}}{\partial{z}} = F_z$$ $$m\ddot{z} + mg = (f_1+f_2) \cos{\theta}$$

*

$$\frac{d}{dt}\left( \frac{\partial{L}}{\partial{\dot{\theta}}} \right) - \frac{\partial{L}}{\partial{\theta}} = M_{\theta}$$ $$J\ddot{\theta} = l \left( -f_1+f_2 \right)$$

State Space Representation

$$X= \begin{pmatrix}x&z&\theta&\dot{x}&\dot{z}&\dot{\theta}\end{pmatrix}^T \qquad U = \begin{pmatrix}f_1 & f_2 \end{pmatrix}^T$$ $$\dot{X} = \begin{pmatrix} \dot{x} \\ \dot{z} \\ \dot{\theta} \\ -\frac{1}{m} \sin{\theta} \left( f_1+f_2 \right) \\ -g + \frac{1}{m} \cos{\theta} \left( f_1+f_2 \right)\\ \frac{l}{J} \left( -f_1+f_2 \right) \end{pmatrix}$$